|
Depth of Field Matters – or HOW FUZZY CAN A
SHARP IMAGE BE?!
An overview lecture in Q&A form Andrew Davidhazy Q. What is meant by circle of confusion? A. When a lens is defocused, an object point gets rendered as a small circle, called the circle of confusion often refered to as c. (Ignoring diffraction.) If the circle of confusion is small enough, the image will look sharp. There is no one circle "small enough" for all circumstances, but rather it depends on how much the image will be enlarged, the quality of the rest of the system, and even the subject. Nevertheless, for 35mm work c=.03mm is generally agreed on as the diameter of the acceptable circle of confusion in the negative. Another rule of thumb is c=1/1730 of the diagonal of the frame, which comes to .025mm for 35mm film. (Zeiss and Sinar are known to be consistent with this rule. Leitz, of Leica fame, used .02 as their limit.) Because most normal focal length lenses are approximately equal to the diagonal of the film format one can sometimes also specify that c = normal focal length / 1730. In a print that is viewed from a distance of 10 inches the allowable diameter for the circle of confusion is about 1/100 inch or 1/4 mm. In the camera the allowable circle must be as many times smaller than this value as the number of times the negative (or digital image formed on the digital sensor) will be enlarged. So, if a 35mm negative is enlarged 8 times to make an 8x12 inch print that will be viewed from 10 inches then the largest circle of confusion in the negative must not exceed a diameter of .03 mm. If the same print will be viewed from a larger distance, let’s say 15 inches, then the diameter of the circle of confusion in the print can grow to .36 mm and in the negative it therefore can achieve a diameter of up to .045 mm. If you make an 11x14 inch print that will be viewed from 10 inches from a 35mm negative then in the negative the circle of confusion must not exceed .023 mm in diameter. If you adjust your viewing distance according to perceiving normal perspective, that is, from a distance determined by multiplying the lens focal length that took the original photograph by the magnification of the enlargement, then the DOF in all your enlargements of the same negative will appear the same. Q. What circle of confusion applies to digital sensors? A. To determine the circle of confusion of any sensor format based on the criterion used for the 35mm cameras divide the circle of confusion for 35mm by the ratio of the linear size of the digital sensor divided into the corresponding film dimension of the 35mm format. For example, if a digital camera has a sensor that 15x22.5 mm it is 1.6 times smaller than a 35mm frame along the same edge. The digital sensor’s circle of confusion must be considered to be .03 mm divided by 1.6 and this is about .018 mm. Ultimatley this means that to achieve a final print size that is the same when you start with a 24x36mm or standard 35mm frame and a 15x22.5mm CCD sensor, the image formed on the CCD or CMOS sensor needs to be blown up 1.6 times farther than what is required of a 35mm frame. Since the CCD image needs to be enlarged more to achieve a particular final print size it needs to have a more stringent allowable circle of confusion. Q. So what are some standardized circles of confusion at the image, film or sensor plane? inches mm 16 mm movie .001 .02 110 (13 X 17 mm) .0012 .03 Digital (15x22.5mm) .0018 .03 but better if .018 135 (24 X 36 mm) .002 .05 but better if .03 2 1/4 Roll film .005 .08 but better if .05 4 x 5-inch and sensor diagonal /1720 critical use larger or film diagonal / 1000 liberal For a 4x5 inch camera with a 6.5 inch diagonal c should not exceed .0037 inches or .09 mm for critical use or .0065 inches or .16 mm for liberal use. The above values are for circles of confusion at image plane on negatives or digital sensors. The acceptable maximum size of the circle of confusion on a final print that is viewed from a distance of about 10 inches is considered to be about 1/100 inch or about .25 mm (1/4 millimeter). As the viewing distance increases the allowable size of the circle of confusion in a print also increases proportionally to the increase in viewing distance. This is why a billboard image seen from a mile away appears quite sharp but when viewed close-up it is obviously fuzzy. Depth of field in the billboard seen from a distance seems much greater than when the same billboard is examined from close-up. Q. What about digital cameras that have smaller sensors than 35mm cameras? A. Ultimately DOF is inversely proportional to format size assuming the use of normal focal length lenses for the given format. The smaller the format the shorter the normal focal length lens used and ultimately the larger the DOF. Dealing mostly with digital cameras that are compared to 35mm film cameras note that since format size is inversely proportional to the "digital multiplier" the higher the "digital multiplier", the smaller the format and thus proportionally the greater the depth of field. The digital multiplier is simply a way of saying that the digital sensor is some value smaller than the standard 35mm format to which the sensor is compared. This is one of the reasons large format camera users need tilts and swings to get adequate depth of field. With an 8x10 camera you have about 8.5 times LESS depth of field than you do with 35mm for the same image. This also explains why digital cameras, some of which have sensors 1/6 the size of 35mm film, have such a large depth of field and one of the reasons why it's almost impossible to get blurred backgrounds when using them. So if you make the same size print and shoot with a lens that gives you the same view and you use the same aperture, if you halve the format size you double the DOF, if you double the format size you halve the DOF. Q. What's the relative DOF if you use the SAME lens on different camera bodies? A. Now you run into the problem of what you are comparing to what. The same lens on the two formats will give you different fields of view, so if you enlarge each image to the same size (say 8x12), you won't have the same print but you can still compare DOFs. If you crop the 35mm negative to give you the same print as the digital image the answer is easy. The DOF in the cropped 35mm print and digital image print will be exactly the same. Essentially you're using the same lens and same size image (cropped 35mm or digital), so you get exactly the same DOF. Q. How does changing focal length affect the perception of depth of field? A. In short, as focal length increases the perceived depth of field decreases. This means there is an inverse relationship between them. But one needs to be a bit more specific. For example, if a 35mm camera and a large format camera are each fitted with their own appropriate normal focal length lenses, such as a 50mm on the 35 mm and a 150 mm on the 4x5) the depth of field in equal sized prints made from the two cameras will show an inverse relationship between focal length and depth of field. The picture made with the 50 mm lens will show 3 times the depth of field as the 150 mm lens. On the other hand, if one uses a 50mm lens and a 150 mm lens on the SAME camera then the situation is more dramatic in outcome with the 150mm lens producing 9 times less depth of field than the 50 mm. This means that the ratio now varies inversely with their focal lengths squared. Since the ratio of their focal lengths is 1:3 the ratio of their focal lengths squared is 1:9. This is the case as long as the negatives made with each lens are enlarged to the same degree. However, if the smaller image made with the 50mm lens is enlarged by moving the camera closer to the subject so that it matches in size that made with the 150mm lens then the apparent depth of field in both cases is the same. The reason for this is that the camera needs to be moved to 1/3 the original distance. Since depth of field increases with the distance squared the new depth of field would be 1/9 th what it was before and so exactly the same as what one would obtain with the 150mm lens. Q. What if I use a shorter focal length lens but blow up the negative to match images sizes between photographs made with a short and a long focal length lens? A. If you photograph a scene with two different focal length lenses (set to the same aperture) and then blow-up the image made with the shorter one so it matches the image made with the longer one then you still do perceive a gain in depth of field although not as great as when the images are not enlarged. In this case depth of field will essentially be simply inversely related to lens focal length. The shorter focal length lens will render a depth of field that is as many times greater than the long focal length lens as the ratio of their focal lengths. In this hypothetical case the 50 mm lens, being 1/3 the focal length of the 150mm lens, will exhibit a depth of field that is three times as great as that which the 150mm lens exhibits. Q. Can I get greater depth of field by moving the camera further from a subject? A. Yes. If you are satisfied with a smaller image resulting from moving further away from a subject then the increase in depth of field is dramatic. Doubling the object distance quadruples the depth of field. However, if you enlarge the negative taken from further away so that the subject size matches that you got from closer up, then you still get an effective increase in depth of field but only proportional to the increase in subject distance. Doubling the subject distance doubles the depth of field. Of course you then also have to deal with an increase in grain size with film or digital noise in a digital system. Q. What is hyperfocal distance? A. The closest distance that is in acceptable focus when the lens is focused at infinity. It assumes that a print will be viewed from a normal viewing distance and this is the camera lens focal length multiplied by whatever degree the print was enlarged to. It depends only on lens focal length, f number and acceptable size of circle of confusion. It can be determined from the following relationship: h = lens focal length squared / (f# times c) Q. What are the closest and farthest points that will be in acceptably sharp focus? There are several ways to determine this. This is one of them. It is a somewhat more precise method than the one mentioned in the M&P book. A. Distance Far = h times U / (h + (U - f)) Distance Near = h times U / (h - (U - f)) Q. What is depth of field? A. It is sometimes convenient to think of a rear or far depth of field and a front or near depth of field. The rear depth of field is the distance from the object to the farthest point from the camera that is considered sharp and the front depth of field is the distance from the closest point that is sharp to the object focused upon. The term “depth of field” is used for the combination of these two, i.e. the distance from the closest point that is sharp to the farthest point that is sharp. That is, the near or front distance considered sharp subtracted from the far distance considered sharp is the total Depth of Field in a photograph. Q. What is Depth of Field Preview? A. It is a feature on higher quality SLR cameras that allows you to stop down the lens while looking though the viewfinder. Ostensibly it allows you to "preview" the depth of field. Of course, since this stops down the aperture, the image also gets dimmer. Most people find it difficult to judge from a dim viewfinder image whether some part of the image will appear sharp in a slide or print. However, in many cases photographers will select a large aperture to deliberately blur background or foreground objects. DOF preview lets you see just what the effect will be. Q. Where should I focus my lens so I will get everything from some close point to infinity in focus? A. At the hyperfocal distance. (More precisely, at Object Distance = h + f ). In this condition the closest part of the scene that will be in focus is at half the distance focused upon. Sometimes this is stated as a definition of the hyperfocal distance. Essentially when depth of field in a photograph extends from some near point just to infinity the lens is focused on the hyperfocal distance and this is twice as far from the camera as the near point sharp. Q. What is and how do you use a DOF scale on a lens? A Depth of Field scale is included on the barrels of many rigid body (SLRs as well as rangefinder models) cameras or lenses to provide the photographer a general idea of what can be expected in terms of depth of field when using various apertures and as an aid to selecting the f# that will provide the greatest depth of field under given conditions. These scales assume that prints will be viewed from normal viewind distances. These are close to the diagonal of an enlargement if the image is not cropped. Or, if the enlargement is cropped then from a distance that the uncropped print would have been viewed from. 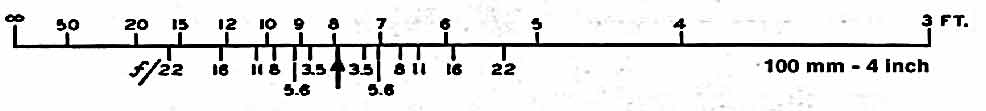 This shows what you might expect to see on a camera lens. It is aguide to determining the depth of field limits of this lens that is sharply focused on a distance of about 8 feet. On either side of the arrow indicating the object in sharp focus you see the camera’s series of f numbers. Note that f/16 on one side of the point of sharp focus is opposite the 12 foot mark while on the right side f/16 appears opposte the 6 foot mark. This means the total depth of field is 12-6 or six feet and the lens is focused on 8 feet. So there is a distance of 2 feet towards the camera within which everything appears sharp and 4 feet beyond the subject fopcused on the will also appear sharp.  In the illustration above the lens is focused at infinity. Note that one can determine what the hypefocal distance is at any selected f# by seeing what distance falls opposite these numbers. For example, at f/16 the near point sharp is 20 feet. At f/22 it is 15 feet. Between f/5.6 and 8 it is 50 feet. If, as shown below, one selects a particular aperture and focuses the lens on the associated hyperfocal distance you can see that the depth of field extends from 1/2 the hyperfocal disstance all the way to infinity. In the case shown, f/16 was selected as the shooting aperture and when one focuses at 22 feet it can be seen that depth of field extends from infinity, the location of one of the f/16 marks, all the way to 11 feet, the location of the second f/16 mark. 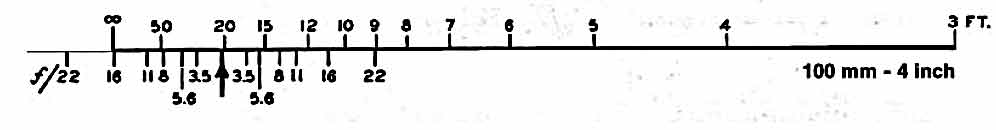 Q. How much will depth of field change if I change from f/11 to f/22? Perceived depth of field will vary directly with the f number. This means that if you got, let’s say, a 5 feet depth of field at f/11 then at f/22 you will perceive exactly twice as much depth of field or 10 feet. To obtain the least possible depth of field use the largest diaphragm setting or aperture possible. If this results in too much light going through to the film or digital sensor and you have exhausted the possibility of shortening your shutter speed then use Neutral Density filters over the lens or resort to a slower film or sensor speed or somehow creatively lower the ambient light level. Q. What do I do to determine Depth of Field if I have a lens that does not have a scale or if I am using a view camera? A. You can compute your own! You need to know what the ( f ) focal length of the lens is you will be using, what the ( D ) distance is that your lens will be focused on (the distance to the subject), and the ( F ) lens aperture you are planning on using and what your permissible ( c ) circle of confusion is on the negative or the enlargement. To do this proceed as follows: Assume you will be shooting at f/16, the object is at 20 feet and you will use a 6 inch (150mm) lens on a view camera. The acceptable size for the Circle of Confusion in this case we will determine from the fact we are shooting on 4x5 inch film whose diagonal is 6.5 inches and so the accepatble diamtere is 6.5 inches divided by 1730 or .0038 inches (or about .1mm). The next thing to do is to determine the Hyperfocal Distance. The formula for HD (Hyperfocal Distance) with any focal length lens at any aperture is given by: HD = f squared / (f# x C) where f is focal length f# is the f-number C is the maximum diameter of the Circle of Confusion HD = focal length squared / f# x dia. of C We assume it (C) to be 1/1730 of 6.5 inches which is the diagonal of a 4x5 inch negative ( so 6.5 / 1730 = .0038 inches) So, HD = 6 inches squared / (16 x .0038 inches) = 36 / (16 x .0038) 36 / .0608 592 inches or about 50 feet To determine the DOF you need to determine the Near Distance and the Far Distance that is reproduced acceptably sharp and this can be determined once the HD or Hyperfocal Distance has been determined. As follows: DN = HD x U / (HD + U) DF = HD x U / (HD - U) Where DN is distance to Near Point in subject reproduced sharply DF is distance to Far Point in subject reproduced sharply U is the distance to the Object that the lens is sharply focused on And finally the total DOF = DF - DN Now, let's digress and assume you focus that lens _on_ the HD and see what happens: DN = 50 ft x 50 ft / (50 ft + 50 ft ) = 2500 / 100 = 25 DF = 50 ft x 50 ft / (50 ft – 50 ft ) = 2500 / 0 = infinity DOF extends from 25 feet to Infinity ! This shows you an important fact regarding the Hyperfocal Distance and that is that when you focus the lens on the Hyperfocal Distance the Depth of Field extends from half the Hyperfocal Distance to Infinity! Now let's change the point on which you focus the lens to 20 feet. What would the DOF be then? DN = 50 ft x 20 ft / (50 ft + 20 ft) = 1000 / 70 = 14.28 DF = 50 ft x 20 ft / (50 ft - 20 ft) = 1000 / 30 = 33.33 DOF extends from 14.28 to 33.33 feet and thus it is 19 feet You can see how once the Hyperfocal Distance has been determined you can use it to easily determine the DOF by finding the Near and Far distances sharp given a particular Subject Distance (U). Q. What is Depth of Focus? A. This is a subject that does not come up for discussion to often but it refers to the accuracy with which the sensor or film plane of the camera matches the sharply focused image plane of the lens. It deals with imprecision in the placement of the film or sensor plane while still recording a subject’s image sharply. Depth of focus is a chracteristic of cameras that has to do with placement of the image receptor at the image plane of the lens. Small discrepancies in accuracy of placement can be tolerated as long as they do not cause the sharply focused image of a subejct to be defocused beyond the limits imposed by the circle of confusion criterion elaborated on above. The utlimate consequence of errors in placement of the film or sensor in relation to the sharply focused image of a given scene is that depth of FIELD assumptions are negated or invalidated. Depth of focus only “guaramtees” that the image of a subject the lens is focused on will not be noticeably unsharp. If an image is focused at the limits of the depth of focus then depth of field in “subject space” will be altered and all the care with which depth of field might have been computed will be invalidated. Q. When is depth of focus of primary concern? A. When you are trying to focus on a 2-dimensional subject such as when doing copy work. Essentially the subject has no depth but the film or digital sensor could be located at several positions and still render an acceptably sharp image. This is the cae so long as the defocusing effect of placing the recording surface at different distances from the lens does not produce changes in the circles of confusion that magnify them to a value larger thanthat acceptable for the particular film/digital format being used. Some general summary points related to the
Depth of Field - FYI
(01) Lenses, unlike
pinholes, can only sharply reproduce a single plane in the subject but pinhole images are equally unsharp everywhere. (02) Out-of-focus image points produced by lenses form discs of light known as circles of confusion. These circles, overlapping, make up unsharp images. (03) Circles of confusion below a certain diameter are acceptable to the eye as points. Maximum permissible size varies with degree of negative enlargement, and distance of viewing: General guide — 1/100 in on the negative divided by expected degree of linear enlargement. .03 mm is often applied to 35mm camera lenses. (04) Enlargements beyond the assumed degree on which the permissible circle of confusion is based will result in less perceived depth of field in the prints if the viewing distance is not proportionally adjusted. (05) Contact prints of film exposed in a camera or a print from a digital camera that matches the camera’s sensor size will exhibit much greater depth of field than an enlargement if viewed from a standard viewing distance of 10”. (06) Permissible movement of the image plane before a subject point appears noticeably unsharp is known as depth of focus. (07) Permissible movement of the subject towards and away from the lens before its image on a focal plane appears noticeably unsharp is known as depth of field. (08) Both depths vary with circle of confusion, f-number, focal length and subject distance, but as image size increases depth of focus increases, depth of field is reduced. Minimum depth of field gives emphasis to a particular plane in the subject, while maximum depth of field gives overall information. (09) Under similar conditions the smaller camera with smaller format film or image sensor produces greater depth of field. (10) Depth of field calculators are used as aids for scale-focused cameras and although depth of field can be visually estimated in SLR camers the “calculator” on many lenses can provide additional information on depth of field limits as estimated by the use the manufacturer assumes the lens will be to. (11) Larger object distances produce greater perceived depth of field in the resulting photographs. However if the larger object distance is overcome by moving the camera closer to the subject of interest so that it matches that made from the closer distance then the depth of field will be similar. If a photograph is taken from a larger distance and the negative enlarged so that images match in size then the one taken from a larger distance will exhibit greater depth of field. (12) Using different focal length lenses on the same camera (at the same aperture) will result in a dramatic difference in perceived depth of field as it is determined by the ratio of each focal length squared. But if the photograph made with the shorter focal length lens is made from a closer distance so that the subject size is the same in both images then the depth of field will be the same in both prints. (13) Doubling the f number brings the hyperfocal distance twice as near to the camera and thus its distance exhibits an inverse relationship to the f number. (14) Larger f numbers (smaller apertures) provide greater depth of field than smaller f numbers (larger apertures). (15) For maximum depth of field stretching back to infinity under given conditions, the lens should be focused on the hyperfocal distance. Depth then extends from infinity to one half the hyperfocal distance. (16) Depth of focus is primarily a concern when photographing 2-dimensional subjects such as documents and flat art. (17) When a small format camera and a large format camera are each equipped with “normal” focal length lenses (focal length about equal to diagonal) the depth of field peceived will be inversely proportional to their focal focal lengths assuming both are enlarged to the same final print size. (18) Depth of focus increases as the subject is photographed from ever closer distances while depth of field decreases. (19) There are three basic controls over depth of field. These are: the f-number, the object distance and the lens focal length. (20) The quality of a lens is generally independent of the size of the circle of confusion on which depth of field tables or calculators are based. (21) If a large circle of confusion is chosen to base a depth of field table on it will seem that large apertures will provide depth of field limits that are only achieved with smaller apertures when the chosen circle of confusion is smaller. (22) Increasing the viewing distance to any print will make it appear as if the depth of field in the depicted scene is greater. Disclaimer: The above material; is not all original. It is a compilation drawn from memory and various sources and it in most instances reworded to fit the objectives of this piece. Anything that is incorrect you can blame on me! This piece was assembled for a lecture on Hyperfocal Distance and Depth of Field given in 2004. It was installed in the list of articles in 2007.
Andrew Davidhazy, andpph@rit.edu
- 2004 |